M Theory Lesson 101
In their paper on trialgebras, Loday and Ronco begin with two operations, left $\dashv$ and right $\vdash$, which can form triple products in eight possible ways as indicated by the vertices of the cube.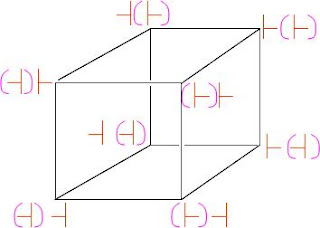 
Note that the three directions correspond to (i) flip first operation (ii) flip second operation or (iii) reorder operations. A third operation $\perp$ is introduced as an interval lying between left and right. For example, $\perp$ may be the associator between left association and right association on three objects, represented by planar binary trees with three leaves.
Loday's later paper on stuffles (also called quasi-shuffles) discusses the MZV algebra in terms of trialgebras. It gives a trialgebra structure to $T(A)$, the tensor algebra for a (commutative) algebra $A$. For the stuffle product $\ast$ the operations left and right are given by
$x \ast y = x \dashv y + x \vdash y + x \perp y$
$ax \dashv by = a(x \ast by)$ with $1 \dashv x = 0$ and $x \dashv 1 = x$
$ax \vdash by = b(ax \ast y)$ with $1 \vdash x = x$ and $x \vdash 1 = 0$
so the left (right) operation has a right (left) unit. The $\perp$ operation is thought of as a deformation of shuffle product. The algebra determined by $\perp$ (with $x \perp y = y \perp x$) and $\dashv$ (with $x \vdash y = y \dashv x$) is a commutative example of trialgebra, leading to the idea that non-commutative zeta values may be studied in the context of trialgebras and their operads. Loday also shows that the functor from commutative algebras to commutative trialgebras of this type has a right adjoint which forgets the operation $\dashv$. Thus this type of algebra forms a natural triple with commutative and associative algebras.
Note that the three directions correspond to (i) flip first operation (ii) flip second operation or (iii) reorder operations. A third operation $\perp$ is introduced as an interval lying between left and right. For example, $\perp$ may be the associator between left association and right association on three objects, represented by planar binary trees with three leaves.Loday's later paper on stuffles (also called quasi-shuffles) discusses the MZV algebra in terms of trialgebras. It gives a trialgebra structure to $T(A)$, the tensor algebra for a (commutative) algebra $A$. For the stuffle product $\ast$ the operations left and right are given by
$x \ast y = x \dashv y + x \vdash y + x \perp y$
$ax \dashv by = a(x \ast by)$ with $1 \dashv x = 0$ and $x \dashv 1 = x$
$ax \vdash by = b(ax \ast y)$ with $1 \vdash x = x$ and $x \vdash 1 = 0$
so the left (right) operation has a right (left) unit. The $\perp$ operation is thought of as a deformation of shuffle product. The algebra determined by $\perp$ (with $x \perp y = y \perp x$) and $\dashv$ (with $x \vdash y = y \dashv x$) is a commutative example of trialgebra, leading to the idea that non-commutative zeta values may be studied in the context of trialgebras and their operads. Loday also shows that the functor from commutative algebras to commutative trialgebras of this type has a right adjoint which forgets the operation $\dashv$. Thus this type of algebra forms a natural triple with commutative and associative algebras.
posted by Kea | 1:50 PM
2 Comments:
Dear Kea,
I am sorry about off topic comment but it is about so shattering experimental discovery that it is my duty to make it.
Plasmoids as primitive lifeforms quantum controlled by dark matter at their "field bodies" is one of basic predictions of TGD inspired quantum biology. For few years ago Romanian group of physicist found evidence for this.
For a week or so ago I learned about mysterious spectral lines and bands in IR and visible region known by astrophysicists but having no identification in terms of chemistry (see this). I realized that they can be identified as lines resulting when electrons or protons drop to larger space-time sheets from smaller ones (zero point kinetic energy defining spectrum of universal metabolic quanta is liberated in the process). These lines are associated with interstellar dust contain PAHs (polycyclic aromatic hydrocarbons, basic candidates for simplest molecules involving electron pairs with hbar= n*hbar0, n=5 or 6): they are believed to be predecessors of our molecular life. The conclusion was this dust is alive in plasmoid sense using UV light with energies above 7.5 eV from stars as metabolic energy: The quanta 4, 6, 8 eV might be important even for some primitive forms of ordinary life.
Yesterday evening I learned that interstellar dust behaves like living matter in some respects!. This is a really shattering finding and with single blow destroys the standard dogma about life as something purely chemical (and visible in microscope,...god grief;-)). It should also give bad headaches for those misguided colleagues who have talked about the necessity to accept anthropic principle in order to guarantee continual funding of string theory;-).
Here is the link.
Plasma phase is involved and the dust life constructs analogs of DNA double helices. This has been achieved also in laboratory. "From dust to dust" seems to have a very deep side meaning!;-).
This is all I know at this moment but it is clear that TGD inspired quantum biology is now making a further breakthrough.
Hi Matti. Sounds very intriguing!
Post a Comment
<< Home